Johannes Kepler – the golden ratio in "World Harmonic"

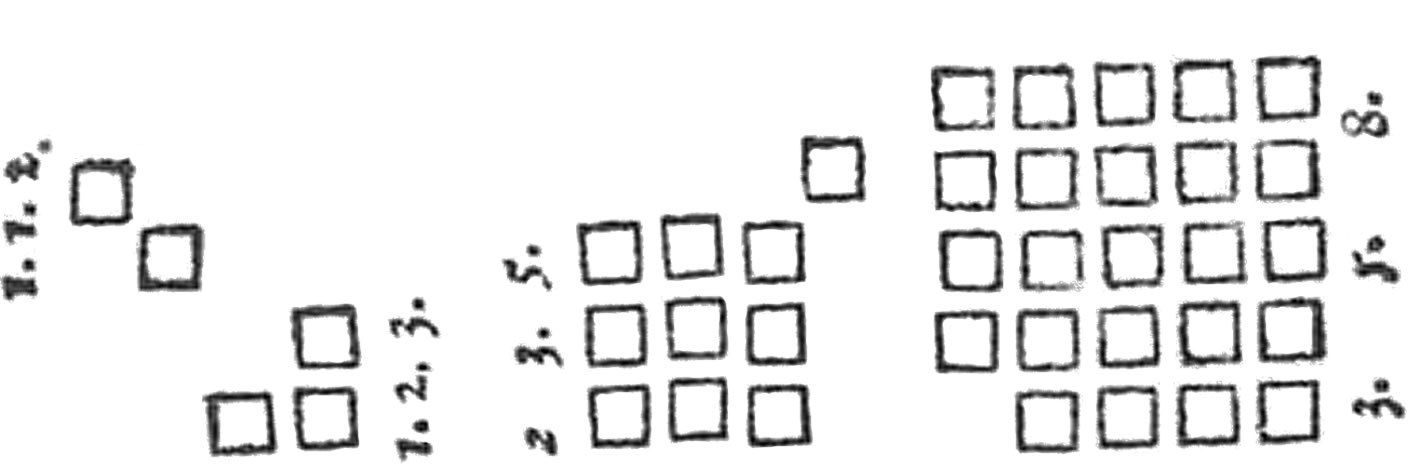
Without referring to Fibonacci, the German astronomer and mathematician Johannes Kepler (1571–1630) also dealt with the number sequence 1, 2, 3, 5, 8, 13, 21 …, albeit in a completely different context.

In his work on World Harmonic, external page "Harmonices Mundi" (1619), Kepler speaks in connection with music of the spheres and the ratios of tone intervals about the golden ratio, the "proportio divina". He finds in this the "beautiful idea of continuing procreation": If the larger part of a line divided by the golden ratio is added to the whole, the total for this line is still in the proportion of the golden ratio. While this proportion cannot be expressed in rational numbers, there is nonetheless a number sequence that gets ever closer to the true value.

Kepler then mentions the first numbers of the Fibonacci sequence (1, 2, 3, 5, 8, 13, 21 …) and explains the principle for forming its elements as the sum of the two preceding numbers, which can be continued to infinity. He further observes that the square of any number in this sequence differs by 1 from the product of the two adjacent numbers, with the difference being alternately positive or negative. Kepler also formulates the approximation of the golden ratio by the proportions of consecutive Fibonacci numbers.