Development of numeral systems
Our present-day way of writing numerals is so self-evident to us that we hardly give it a second thought. A glance into history shows what immense advantages the decimal place-value system offers compared with other numeral systems.
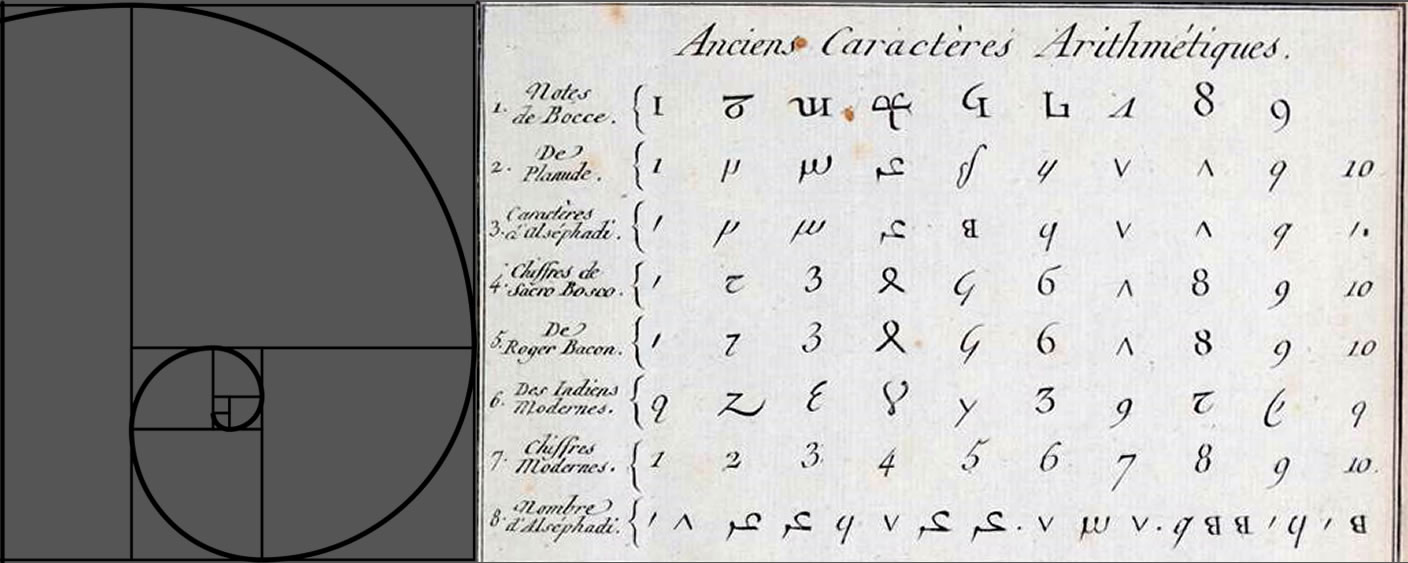
Numeral systems of the Babylonians, Greeks and Romans
The Babylonians were already familiar with a place-value system at the beginning of the second millennium BCE: on the basis of the old Sumerian sexagesimal system, they wrote all numbers with only two cuneiform characters, which meant – depending on their position – one, ten or sixty. The Babylonian way of writing numerals already enabled highly developed algebra and complicated calculations. Compared with this, the Greek and Roman ways of writing numerals were a step back.
The Greeks used all the letters of the alphabet to represent ones, tens and hundreds. While the Romans had fewer numerals, numbers were nonetheless formed likewise purely by addition of the corresponding numerical values. Written calculations were almost impossible using this method. People got by with an abacus on which small stones were placed in parallel columns that showed the decimal place-value. So although the principle of the place-value system was known, it lacked the corresponding way of writing numerals.
Precursors of our present-day numbers
Such a way of writing numerals with the precursors of our present-day digits 1 to 9 had been developed in India by the middle of the sixth century CE. And zero – first written as a dot then as a circle – emerged in written documents about this time. This numeral system enabled any large number to be represented, and calculations could finally be performed easily. In the eighth century the Arabs adopted – via trading routes – the Indian numeral system and the corresponding calculating methods. Knowledge of these spread to Europe in the tenth century.
First systematic representation of the decimal system
Fibonacci provided the first systematic representation with his "Liber abaci". The Italian trading cities furnished a particularly favourable breeding ground for the new calculating method. But, despite all the advantages, calculating with Indo-Arabic numerals was only reluctantly implemented in everyday practice. Arabic numbers were regarded as insecure against forgery as a zero could easily be turned into a 6 or a 9. Published in 1522, the external page "Rechenpüchlein" (Little calculating book) by Jakob Köbel further based on "German" – i.e. Roman – numerals since they were easier for laymen to learn.